

3D Modelling and Camera Geometry
Many 3D Modelling software packages can be used, but the software must be able to deal with:
- 1. Use of real world co-ordinates and mathematical re-projection from the local projection system to the ellipsoid.
- 2. Camera rendering settings which allow for either planar or cylindrical re-projection of the images.
- 3. Curvature of the earth from viewpoints over 1km distance from the development.
3D Modelling is relatively straightforward, even for complex models. 3D Models must be constructed in metres in real world co-ordinates.The critical factor is to ensure the camera is positioned accurately in the view; once achieved, the same camera settings can be input into the 3D modelling software and used to create a precise render. For planar images, it is important that the precise horizontal angle of the view is known.
Even with a 50mm prime lens there is a variable focal length between both ends of the focal range of the lens, so it is correct to say that no lens is precisely 50mm: it will normally be somewhere between 47mm and 52mm. It is essential that you can calculate precisely what that focal length is.
Once the precise focal length is known, the following equation will give you the precise horizontal angle of view:
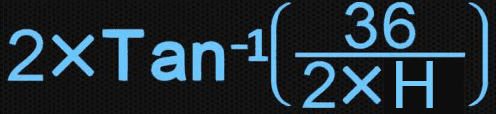
Once you know the precise horizontal angle of view you can use this in your 3D Modelling software’s camera geometry render settings.
You also need to know the precise direction of the centre of the image. Whilst a compass on-site will help, this must be precise in order to ensure that the actual camera/image direction and the 3D Model camera line up.
You can then enter the same pixel dimensions (e.g. 5760 x 3840) into the render dimensions. This will achieve the same scaling between the camera image and the 3D model render.







Laser Rangefinders
Where LIDAR DSM data is unavailable Laser Rangefinders can be used to capture real-time positions on buildings. This allows highly accurate points to be entered into the 3D model to correspond with the actual photograph. These might typically include ridge lines and eaves of neighbouring buildings or telegraph poles.





